Determining Minimum Sightlines at Grade Crossings

A Guide for Road Authorities and Railway Companies

Part One: Overview

1.1 Purpose

This Guide contains advice and technical guidance that stakeholders (road authorities, private authorities and railway companies) need to determine the minimum sightlines required at grade crossings.

The Grade Crossings Regulations (GCR) and Grade Crossings Standards (GCS) were introduced on November 28, 2014. They require road authorities and railways to establish and maintain sightlines at grade crossings. These sightlines must, at minimum, provide crossing users with enough time to see and react to an oncoming train, from both the ‘approach' and ‘stop' positions.

This Guide replaces the Minimum Railway/Road Crossing Sightline Requirements for All Grade Crossings without Automatic Warning Devices G4-A. While you may still use the G4-A guideline as a quick reference guide, the sightline requirements in this Guide are based on the new Grade Crossings Regulations and Grade Crossings Standards, so are more accurate, flexible and descriptive.

In addition to this Guide, you should have a thorough knowledge of the key documents that specify the design guidelines and standards for grade crossings, including:

• Grade Crossings Standards (GCS)
• Grade Crossings Regulations (GCR)
• Geometric Design Guide for Canadian Roads by the Transportation Association of Canada (TAC); and
• TAC's Manual of Uniform Traffic Control Devices for Canada (MUTCDC).

1.2 A Phased-in Approach

The Grade Crossings Regulations use a phased-in approach providing flexibility. In November, 2021, changes were made to the regulations’ compliance deadlines. The compliance timelines have been modified in a manner that prioritizes grade crossings by levels of risk and the requirements for sightlines are as follows:

• November 28, 2022 for high-priority grade crossings, and
• November 28, 2024 for all other grade crossings

Sightline requirements in the GCR and GCS must be in place at:

• all new crossings when they are built or significantly altered (see GCR, section 20), and
• all other crossings based on a grade crossings level of risk.

Learn how these amendments could affect you

More information on the latest changes to the Grade Crossings Regulations

1.3 Application

The minimum sightline requirements set out in GCR sections 20, 21 and 22; and in GCS, article 7; enable grade crossing users to safely see and react to an oncoming train. These requirements apply to all public and private grade crossings.

For the purpose of defining sightlines, every crossing has four quadrants created by the angle formed by the intersection of the road and the track. You must determine minimum sightlines for all four quadrants of the crossing so crossing users can see an oncoming train from both road approach directions while they are in the ‘approach' and the ‘stop' positions. For increased safety, Transport Canada strongly encourages you to provide sightlines above and beyond the minimum requirements identified in this guide, as a safe crossing is a visible crossing.

In addition to establishing unobstructed sightlines, you must:

• keep sightlines clear of trees, brush and stored materials to protect the visibility of the grade crossing, railway crossing warning signs, signals, and approaching trains; and
• ensure that highway traffic signs, utility poles and other roadside installations do not obstruct the view of railway crossing signs, signals and warning systems.

1.4 Variables to Consider

In some cases, increasing minimum sightlines to account for factors affecting the acceleration or deceleration of vehicles using the road may be required. Such factors include road gradient and surface condition as well as vehicle weight, length and power.

Notes:

• (1) If the road crossing design speed or the railway design speed differs on either side of the grade crossing, you must make stand-alone calculations for each quadrant.
• (2) Take sightlines for drivers stopped at a grade crossing from a position no closer than 5 meters from the nearest rail, measured from the driver's position in the vehicle.

1.5 Collaboration of Authorities

The GCR Information Sharing requirements, encourage railway companies and road authorities to work together to meet sightline requirements. For example:

• The railway company is responsible for providing the road authority with its railways design speeds and train volumes.
• The road authority is responsible to provide the railway company with the road crossing design speeds and the design vehicle using the grade crossing.

Since both the railway company and road authority are responsible for providing and maintaining adequate sightlines for their infrastructure, it is very important that both:

• Are aware of all factors affecting sightlines; and ensure that any changes to these are relayed to either party immediately.

1.6 Flexible Options

The GCR provide flexibility for achieving minimum sightlines and can be adapted to the unique physical and operational attributes of each crossing. For example, for crossings without a grade crossing warning system, you can achieve minimum sightlines by clearing sightline obstructions, or reducing train or vehicle speeds. In some cases you can restrict the use of heavy or long combination vehicles, or improve road approach gradients. Other options may include installing a STOP sign or Active Warning System^{Footnote 1}.

• Requirements for sightlines at grade crossings without a grade crossing warning system are specified in GCR subsections 20(2) and 21(1), which refer to GCS figures 7-1 (a) and (b).
• Requirements for sightlines at grade crossings with a grade crossing warning system are specified in subsections 20(1) and 21(2), which refer only to GCS figure 7-1 (a).

1.7 Exceptions and Additional Sightline Requirements:

Sightline requirements vary depending on the safety attributes at the grade crossing:

• Public or Private grade crossing with a Warning System with Gates: sightline requirements do not apply but the warning system must be visible throughout the Stopping Sight Distance (SSD).
• Public or Private grade crossing with STOP sign or Warning System: sightlines are required from the 'stop' position only, as shown in Figure 3 from section 2.2.2 of this Guide. The STOP sign and Warning System must be visible throughout the SSD.
• Private grade crossing where the railway design speed is 25 km/h (15 mph) or less and access to the road leading to the grade crossing is controlled by a locked barrier, or the grade crossing is for the exclusive use of the private authority and is not used by the public: sightline requirements do not apply (however, it is strongly encouraged to provide sightlines at all times); and
• Public or private grade crossing being operated under Manual Protection or sometimes referred to as STOP and Proceed (where the road users are stopped by a flag person and the railway equipment must STOP before Proceeding over the crossing): sightline requirements are limited to visibility of the grade crossing throughout the SSD.

Part Two: Calculating Sightlines

If the road crossing speed or the railway design speed varies on either side of the grade crossing, you must do stand-alone calculations for each quadrant.

2.1 What you need to know:

To establish the minimum sightlines for each quadrant of any grade crossing you must first determine six key factors. Doing this in advance will make the sightline calculation process easier.

Factor 1: Design vehicle and its dimensions

A ‘design vehicle' is the most restrictive vehicle that authorities expect to routinely use the grade crossing:

• The road authority chooses the design vehicle for a public grade crossing.
• The railway company chooses the design vehicle for a private grade crossing.

There are three classes of design vehicle: 1) Passenger Cars; 2) Trucks; and 3) Buses. Longer or larger vehicles usually generate a larger clear sightline triangle. See Table 1 below for vehicle descriptions.

Table 1 – Design vehicle Lengths/Class

General Vehicle Descriptions	Length (m)	Design Vehicle Class
Passenger Cars, Vans and Pickups (P)	5.6	Passenger Car
Light Single-unit Trucks (LSU)	6.4	Truck
Medium Single-unit Truck (MSU)	10.0	Truck
Heavy Single-unit Truck (HSU)	11.5	Truck
WB-19 Tractor-Semitrailers (WB-19)	20.7	Truck
WB-20 Tractor-Semitrailers (WB-20)	22.7	Truck
A-Train Double (ATD)	24.5	Truck
B-Train Double (BTD)	25.0	Truck
Standard Single-Unit Buses (B-12)	12.2	Bus
Articulated Buses (A-BUS)	18.3	Bus
Inter-city Buses (I-BUS)	14.0	Bus

Source: Geometric Design Guide for Canadian Roads, TAC; September 1999.

Note: Table 1 is a list of the design vehicles, vehicle classes and their dimensions that are in regular operation on Canadian roads and are referenced in the GCR. However, there are four categories of Special Vehicles referenced in the TAC Geometric Design Guide (but are not included in Table 1). These are; Long Load Vehicles, Long Combination Vehicles, Towed Recreational Vehicles, and Large Trucks with Tandem or Triple Steering Axle that may be selected to address special traffic operational conditions or for restricted vehicle routes. Should a Special Vehicle be chosen as the design vehicle, Road Authorities should obtain the design dimension data directly from the manufacturers for specific Special Vehicles.

Design Vehicle Length (L):_______ (m)     Design Vehicle Class: ____________

Factor 2: Road Crossing Design Speed

The ‘road crossing design speed' is the motor vehicle speed that corresponds to the grade crossing's current design:

• The road authority chooses the road crossing design speed for public grade crossings.
• The railway company chooses the road crossing design speed for private grade crossings.

Road Crossing Design Speed (V): _____________ (km/h)

Factor 3: Railway Design Speed

The ‘railway design speed' is the railway equipment speed that corresponds to the grade crossing's current design. The railway company chooses the railway design speed.

Railway Design Speed (V_t): _____________ (mph)

Factor 4: Road Approach Gradient within SSD

The ‘road approach gradient’ (in percentage) is the measurement of the average gradient within the Stopping Sight Distance (SSD). The road approach gradient is always measured in the same direction approaching the crossing from the start of SSD. A positive (+) slope represents an ascending slope and a negative (-) slope represents a descending slope. The road approach gradient must be determined for each road approach. The road authority determines the road approach gradient.

Road Approach Gradient within SSD: ____________ (%)

Factor 5: Stopping Sight Distance - (Table 2)

The Stopping Sight Distance (SSD) is the minimum sight distance required along the road approach for a crossing user to react to approaching railway equipment. The SSD is based on the road crossing design speed and Table 2 below may be used as a quick reference to determine the SSD:

Table 2 – Determine SSD

Road Crossing Design Speed (V) (km/hr)	Stopping Sight Distance (SSD) (m)
	Road Approach Gradient
	-10%	-9%	-8%	-7%	-6%	-5%	-4%	-3%	-2%	-1%	0%	1%	2%	3%	4%	5%	6%	7%	8%	9%	10%
10	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8	8
20	21	21	21	21	21	21	20	20	20	20	20	20	20	20	20	20	19	19	19	19	19
30	33	33	32	32	32	31	31	31	30	30	30	30	30	29	29	29	29	29	29	28	28
40	51	50	49	49	48	48	47	46	46	45	45	45	44	44	43	43	43	42	42	42	42
50	76	75	73	72	71	70	69	68	67	66	65	64	63	63	62	61	61	60	60	59	59
60	104	101	99	97	95	93	91	89	88	86	85	84	83	81	80	79	78	77	77	76	75
70	140	135	132	128	125	122	119	117	114	112	110	108	106	105	103	101	100	99	97	96	95
80	182	176	171	166	161	157	153	149	146	143	140	137	135	132	130	128	126	124	122	121	119
90	223	216	209	202	197	191	186	182	178	174	170	167	163	160	157	155	152	150	148	145	143
100	281	271	262	253	245	238	232	226	220	215	210	205	201	197	194	190	187	184	181	178	175
110	345	331	318	307	296	287	278	270	263	256	250	244	239	234	229	224	220	216	212	209	205

Notes:

1. This table may be used as a guide reference for all design vehicle classes in Table 1 (Geometric Design Guide for Canadian Roads, TAC; September 1999.
2. Table 2, was generated using the formulas contained in article 7.2 of the Grade Crossings Standards.

Stopping Sight Distance (SSD):_______________ (m)

Factor 6: Grade Crossing Clearance Distance

The ‘grade crossing clearance distance' is the distance between the departure point before crossing the tracks, to the clearance point on the other side, away from the conflict zone. Once onsite, or from plans, estimate the grade crossing clearance distance as shown in Figures 1(a) and 1(b)

Figure 1 - Grade Crossing Clearance Distance

• (a) For Grade Crossings with a Warning System or Railway Crossing Sign

 

• (b) For Grade Crossing without a Warning System or Railway Crossing Sign

 

Grade Crossing Clearance Distance (cd): ________ (m)

Once you have determined the six key factors above you can begin to calculate the minimum required grade crossing sightlines as described in section 2.2 below.

2.2 How to Calculate Sightlines

The following process to calculate sightlines in accordance with the Grade Crossings Regulations applies to all grade crossings required to meet the sightline requirements:

• new or existing,
• public or private,
• with or without a grade crossing warning system.

Exceptions to these requirements are listed in section 1.7 above.

To satisfy the GCR, you must use the 6 key factors determined above, to calculate the minimum required sightlines for both the SSD approach point and the ‘stop’ position.

2.2.1 Determining Sightlines from the SSD Approach Point

The SSD is the minimum sight distance along the road approach that a crossing user needs to react to approaching railway equipment. The SSD is based on the road crossing design speed. The method to determine SSD is described in Factor 5 of Section 2.1 of this document.

D_SSD is the minimum distance along the line of railway (in both directions) that a crossing user needs to see approaching railway equipment from the SSD point.

Figure 2 – Minimum Sightlines for Drivers Approaching a Grade Crossing

 

Determining minimum sightlines from the SSD approach point is a two-step process. The values determined in Steps 1 and 2, described below, define the minimum clear sightline area required for the SSD approach position, as indicated in Figure 2 above. This value may be different for each road approach. Although Figure 2 illustrates only one quadrant, you must determine the clear sightline area for all four quadrants (i.e. to the right and left sides of each road approach).

Step 1

Calculate the Minimum Stopping Sight Distance Time (T_SSD) for each road approach to the grade crossing using the formula below.

Formula: T_SSD = [(SSD + cd + L) / (0.278 x V)]

Where :

V = road crossing design speed (km/h)

cd = grade crossing clearance distance (m)

L = length of grade crossing design vehicle (m)

SSD = stopping sight distance from Table 2 based on the design vehicle class (m)

Road approach 1 T_SSD = ________ (s)

Road approach 2 T_SSD = ________ (s)

Step 2

Calculate the Minimum Sightlines along the Rail Line (D_SSD) for each road approach using Table 3 OR the formula indicated below Table 3.

Note: To use Table 3, you must:

1. Calculate the T_SSD (see Step 1) for the design vehicle required for the grade crossing, and to determine the railway design speed (V_t) (mph).
2. Select the horizontal line in the Table corresponding to the railway design speed,
3. Move to the right to the column under the T_SSD required for the crossing.

Repeat these steps for each road approach.

Note: If the road crossing design speed or the railway design speed varies on either side of the grade crossing, you must do stand-alone calculations for each quadrant.

Table 3 – Minimum Sightlines along the Rail Line (D_SSD) (as illustrated in Figure 2)

Railway Design Speed Vt (mph) WARNING: Railway design speed in mph!	Stopping Sight Distance Time TSSD (seconds)											If TSSD > 20 sec., add for each additional second (m)
	≤ 10*	11	12	13	14	15	16	17	18	19	20
	Minimum Sightlines along Rail Line (DSSD) (m)
STOP	30	30	30	30	30	30	30	30	30	30	30	+0
1-10	45	50	55	60	65	70	72	76	80	85	90	+5
11-20	90	100	110	120	125	135	145	155	165	170	180	+10
21-30	135	150	165	175	190	205	215	230	245	255	270	+15
31-40	180	200	220	235	250	270	285	305	325	340	360	+20
41-50	225	250	270	290	315	335	360	380	405	425	450	+25
51-60	270	300	325	350	380	405	430	460	485	510	540	+30
61-70	315	350	380	415	445	470	505	535	565	595	630	+35
71-80	360	395	435	465	505	540	580	610	650	680	720	+40
81-90	405	445	490	535	570	605	650	685	730	765	810	+45
91-100	450	500	540	580	630	670	715	760	805	850	895	+50

* Note: When T_SSD is less than 10 seconds, you must use the formulas below, to calculate D_SSD.

You may use the formula below as an alternative to Table 3:

Formula: D_SSD = 0.278 x V_t x T_SSD (m) ** Convert mph –> km/h: mph x 1.6 **

Where :

V_t = railway design speed (km/h)

T_SSD = [(SSD + cd + L) / (0.278 x V)] Stopping Sight Distance Time (from Step 1) (s)

Road approach 1 D_SSD = ________ (m) (applicable to both sides of road approach if V_t similar)

Road approach 2 D_SSD = ________ (m) (applicable to both sides of road approach if V_t similar)

2.2.2 Determining Sightlines from the ‘Stop’ Position

D_stopped is the minimum distance along the line of railway that a crossing user must be able to see approaching railway equipment, from the ‘stop’ position at a grade crossing.

To establish D_stopped, you must:

1. Determine the distance to travel during acceleration over the grade crossing.
2. Use the acceleration curves below to establish the acceleration time of the design vehicle.
3. Use the acceleration time to establish the time required for the design vehicle, or the pedestrian/cyclist/assistive device, to safely clear the crossing (T_stopped).
4. Use the greater of the two values in step 3 to determine the D_stopped measurement needed for sightlines from a ‘stop’ position.

Figure 3 - Minimum Sightlines for Drivers stopped at a Grade Crossing

 

Determining minimum sightlines from the ‘stop’ position is a six step process. The values determined in Steps 1 to 6 below define the minimum clear sightline area required for the ‘stop’ position, as indicated in Figure 3 above. This value may be different for each road approach. Although Figure 3 illustrates only one quadrant, in the field, you must verify the clear sightline area for all four quadrants (i.e. to the right and left sides of each road approach).

Step 1

Calculate distance to travel during acceleration (s) using the formula below:

Formula: s = cd + L, where:

• cd = grade crossing clearance distance (m) (see Figure 1)
• L = length of grade crossing design vehicle (m) (see Table 1)

s = __________ (m)

Step 2

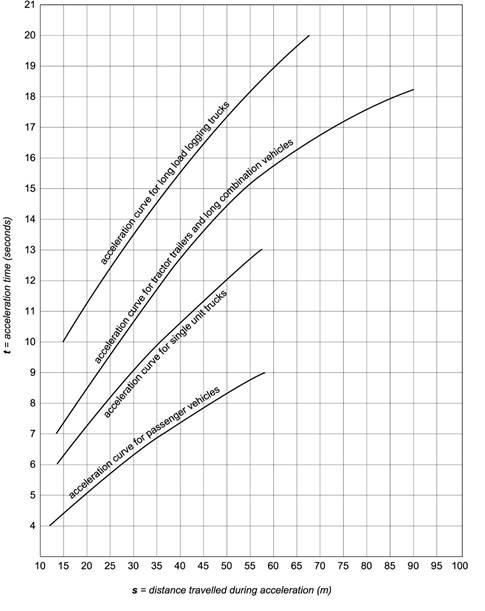
Determine the acceleration time (t) from Graph 1 below:

Graph 1 - Acceleration Curves

 

Source: Geometric Design Guide for Canadian Roads, Transportation Association of Canada; September 1999.

Note: For Design Vehicles not represented in this Graph, you may perform tests or estimate the acceleration time.

t = __________ (s)

Step 3

Calculate the Design Vehicle Departure Time (T_D) for each road approach direction using the formula below:

Formula: T_D = 2 + (t x G) *, where:

• G = ratio of acceleration times based on the gradients from Table 4, below. Road gradient in Table 4 is the most restrictive gradient (or most positive*/least negative gradient*) over the distance that the design vehicle must traverse starting from the rear of the design vehicle, when at the ‘stop’ position, to a point where the rear of the design vehicle passes the clearance point. This value can be different for each road approach to the grade crossing. The road approach gradient at the ’stop’ position is always measured in the same direction approaching the crossing from the ‘stop’ position.  A positive (+) slope represents an ascending slope and a negative (-) slope represents a descending slope. For the purpose of calculating the Design Vehicle Departure Time, the road approach gradient, from a ‘stop’ position, should be determined for each road approach. The value used for G should be the most restrictive gradient (or most positive/least negative gradient value) of the two approaches.
  • Note 1:
    *“Least negative” is defined as the negative value that is closest to zero.
    *“Most positive” is defined as the positive value that is farthest from zero.
  • Note 2:
    For one-way roads, use the actual maximum road gradient (+ or -) within the vehicle travel distance where; S = cd + L for departure time calculations from the stop position.

Road Gradient Effect

For the purpose of calculating the Design Vehicle Departure Time (T_D), adjustments can be made to the acceleration time for a design vehicle on level ground by multiplying the acceleration time by a constant ratio, determined in Table 4 which relates to the most restrictive gradient (or most positive/least negative gradient value) of the two approaches, used for G above.

Ratios for increasing or decreasing the acceleration time along continuous grades of 2% and 4% are provided in the Geometric Design Guide. Table 4 below, is reproduced in the Grade Crossings Standards - Table 10-1 Ratios of Acceleration Times on Grades.

• t = acceleration time from Step 2 (s)

Table 4 - Ratios of Acceleration Times on Grades

Grade Crossing Design Vehicle	Road Grade (%)
	-4	-2	0	+2	+4
Passenger Car	0.7	0.9	1.0	1.1	1.3
Single Unit Truck & Buses	0.8	0.9	1.0	1.1	1.3
Tractor-Semitrailer	0.8	0.9	1.0	1.2	1.7

Source: Geometric Design Guide for Canadian Roads, Transportation Association of Canada; September 1999.

Note: For Design Vehicles not represented in this Table, you may perform tests or estimate the ratio of acceleration times on grades.

Each road approach to a crossing may have a different gradient, departure time, and may therefore require different sightline distances over D_stopped. Accordingly, gradient measurements and departure times must be calculated for all road approaches for conventional multi-direction roads (and once for one-way roads).

Departure time (T_D) from the ‘stop’ position: The total time, in seconds, the design vehicle must travel from the ‘stop’ position to pass completely through the Clearance Distance (cd), is calculated using the following formula:

T_D = J + T

Figure 4 - Profile of Road Approach & Crossing Surface

 

* You may consider adding more time to the calculated time, in accordance with the Acceleration Curves of Graph 1, to account for reduced acceleration caused by the crossing surface, taking into account the number of tracks, surface roughness, super-elevation of the tracks, any unevenness created by the crossing angle, any restrictions on shifting gears while crossing tracks.

Road approach 1 T_D = ________ (s)

Road approach 2 T_D = ________ (s)

Step 4

Calculate Departure Time for Pedestrians, Cyclists and Persons using assistive Devices (T_p), using the formula below:

Formula: T_P = cd/V_p, where:

• cd = grade crossing clearance distance (m)
• V_p = The average travel speed, in metres per second (m/s), for pedestrians, cyclists and persons using assistive devices (to a maximum value of 1.22 m/s)

T_P =____ (s)

Step 5

Determine the departure time (T_stopped) for each road approach using the formula below:

Formula: T_stopped = the greater of the departure times (T_D or T_P)

Road approach 1 T_stopped =______ (s)

Road approach 2 T_stopped =______ (s)

Step 6

Calculate D_stopped for each road approach using Table 5 OR the formula indicated below Table 5, using T_D and T_P for both options.

Note: You must:

1. Calculate the departure time for the design vehicle or the pedestrian/cyclist (T_D or T_P) (from Step 5) required for the crossing and to determine the railway design speed (V_t) (mph).
2. Select the horizontal line in Table 6 corresponding to the railway design speed,
3. Move to the right to the column under the T_stopped (greater of T_D or T_P).

Repeat this process for each road approach.

Note: If the road design speed or the railway design speed varies on either side of the grade crossing, you must do stand-alone calculations for each quadrant.

Table 5 - Minimum Sightlines along the Rail Line (D_stopped) (as illustrated in Figure 3)

Railway Design Speed Vt (mph) WARNING: Railway design speed in mph!	Tstopped = Departure Time (greater of TD or TP) (seconds)											If greater of TD or TP > 20 sec., add for each additional second (m)
	≤ 10	11	12	13	14	15	16	17	18	19	20
	Minimum Sightlines along Rail Line (Dstopped) (m)
STOP	30	30	30	30	30	30	30	30	30	30	30	+0
1-10	45	50	55	60	65	70	72	76	80	85	90	+5
11-20	90	100	110	120	125	135	145	155	165	170	180	+10
21-30	135	150	165	175	190	205	215	230	245	255	270	+15
31-40	180	200	220	235	250	270	285	305	325	340	360	+20
41-50	225	250	270	290	315	335	360	380	405	425	450	+25
51-60	270	300	325	350	380	405	430	460	485	510	540	+30
61-70	315	350	380	415	445	470	505	535	565	595	630	+35
71-80	360	395	435	465	505	540	580	610	650	680	720	+40
81-90	405	445	490	535	570	605	650	685	730	765	810	+45
91-100	450	500	540	580	630	670	715	760	805	850	895	+50

* Note: When T_stopped is less than 10 seconds, you must use the formulas below, to calculate D_stopped.

As an alternative to Table 5, the corresponding formula may be used:

Formula: D_stopped = 0.278 V_t x T_stopped ** Convert mph –> km/h : mph x 1.6 **

Where:

• V_t = railway design speed (km/h)
• T_stopped = departure times as calculated in Step 5 (s)

Road approach 1 D_stopped = ________ (m) (applicable to both sides of road approach if V_t similar)

Road approach 2 D_stopped = ________ (m) (applicable to both sides of road approach if V_t similar)

This concludes the process for calculating minimum sightlines at grade crossings in accordance with the Grade Crossings Regulations (GCR) and Grade Crossings Standards (GCS).

2.3 Next Steps

For increased safety, Transport Canada strongly encourages you to provide sightlines above and beyond the minimum requirements identified in this guide.

The GCR provide flexibility for achieving sightlines and can be adapted to the unique physical and operational attributes of each crossing.

For example, if minimum sightlines cannot be achieved you have the option of clearing the sightline obstructions, or reducing train and/or vehicle speeds, improving road approach gradients/road approach geometry or installing a STOP sign or an Active Warning System (see sections 1.6 and 1.7 of this guide).

2.4 Learn More

If you have questions or want to learn more about sightlines at grade crossings, please contact us by email or phone.

Email: railsafety@tc.gc.ca
Phone: 613-998-2985